Coyote Blog

My son is being recruited, at a minimum, at Bowdoin, Vassar, Wesleyan, Haverford, Kenyon and possibly Amherst and Pomona to play baseball.  We have a pretty good handle on all these schools except Wesleyan in Connecticut, which we have visited but we are having a hard time getting a read on.

In the 2011 Insider's Guide to the Colleges, Wesleyan is described as an extreme example of a college dedicated to politically correct intolerance.  The book says that the classes tend to be mainly focused on teaching kids to be radical activists rather than any traditional subject matter.  Social life is portrayed as revolving around marijuana and hallucinogens.   It is by far the most negative review we have read (well, I suppose this would not be negative to some).

We are trying to get a read on the accuracy of this.    Any of you know this school or attend it?   Is there truth to this, or does the writer have an ax to grind?   He is not naive to what he will find politically at New England liberal arts colleges. The question is not whether there is a lot of leftish political correctness - that is a baseline in all such schools.  The question is whether this school is unusually extreme.  The book makes it sound like it is Kos Kidz Academy.    Comment or send me an email.

Update:  Hmm, based on the comments, I explained myself poorly.  Nic will likely never play pro ball.  If that were his goal, we would definitely be looking to ASU or Texas.  He has decided he wants to go to a small liberal arts college.  Baseball has two synergies - one, he would like to play in college.  Two, being recruited for sports helps in the admissions process at selective schools.

There is money set aside to pay for college, from a source such that it needs to be used for college, so arguments about price-value issues with college are not immediately relevant.

I have written before that peer review is not a guarantee of correctness.  Most academics would laugh at that portrayal, yet that is exactly how climate peer review is treated in the media.

A number of years ago, Charles Monnett, flying over the Arctic to do some sort of whale study, saw 3-4 polar bears floating dead in the water.  Without either a) retrieving the bear carcasses or b) even getting a picture of them, he wrote up a paper that discussed the siting and hypothesized the bears drowned in a storm and further that more bears would likely drown in the future if global warming melts more Arctic ice in the summer.  The findings were the basis for a lot of worry about polar bears, and played a key role in Al Gore's movie.   Panic over the dead bears and Monnett's wild hypotheses about them helped fuel calls for declaring the bears endangered, despite all evidence that their populations have actually been increasing over the last few years.  Monnett did quite well from the work, parlaying his fame into management of a $50 million study budget, the dream of all academics.

Monnett's study has come back into the news because there has been some kind of investigation of him and his work by the Feds.  There has been a lot of speculation among skeptics that the investigation focuses on academic fraud, but I thought that a stretch.  As I wrote here

1. If you read between the lines in the news articles, we really have no idea what is going on.  The guy could have falsified his travel expense reports
2. The likelihood that an Obama Administration agency would be trying to root out academic fraud at all, or that if they did so they would start here, seems absurd to me.
3. There is no room for fraud because the study was, on its face, facile and useless.  The authors basically extrapolated from a single data point.  As I tell folks all the time, if you have only one data point, you can draw virtually any trend line you want through it.  They had no evidence of what caused the bear deaths or if they were in any way typical or part of a trend — it was all pure speculation and crazy extrapolation.  How could there be fraud when there was not any data here in the first place?  The fraud was in the media, Al Gore, and ultimately the EPA treating this with any sort of gravitas.

Seriously, you see four floating bear bodies from 1500 feet, once.  You don't have any facts about how they died.  You only have one data point in time.  Where is there room for fraud?  It's one freaking useless data point.    Here is just a taste of what a joke this study was:

The actual survey Monnett was conducting when he observed the dead bears in 2004 was the migration of bowhead whales.  Investigators questioned how he later obtained data for a table listing live and dead polar bear sightings from 1987 to 2004.

“So how could you make the statement that no dead polar bears were observed” during that time period? May asked.

“Because we talked to the people that had flown the flights, and they would remember whether they had seen any dead polar bears,” Monnett said.

They only mystery is how this unbelievably trivial piece of work was published.

Well, now we have a better idea.  The reviewers for the article were Lisa Rotterman and Andrew Derocher.   Incredibly, it turns out Ms. Rotterman is his wife - yes, some people are more peers than others - and Derocher was awarded a large research contract by Monnett just before he reviewed the article.  Wow.

By the way, I think I will be both right and wrong.  I was pretty sure any government investigation would be about misuse of funds, and that does seem to be the main thrust here, though I was wrong in that it does seem to touch on academic fraud as well, in particular the idea of giving out grant money as a quid pro quo for a positive review  (a practice that skeptics have long sustpected in the climate community).

By the way, both Monnett and his partner Gleason now are claiming that everyone blew their study out of proportion and it wasn't really about global warming.  If this is true, they were sure silent about this when they were basking in all kinds of attention and press and grant money.  Either of them could have stepped forward and stopped the momentum that built from this article and they did not.

By the way, for those who still want to believe that the EPA is drive by science,

Gleason concedes that the study had a major impact on the controversial listing of the bear as an endangered species because of global warming.

“As a side note, talking about my former supervisor, he actually sent me an e-mail at one point saying, ‘You’re the reason polar bears got listed,’” Gleason said.

One sighting in history of four floating dead polar bears and suddenly our whole fossil fuel economy has to be shut down.

Often we libertarians think of making common cause with liberals on social and civil rights issues while making common cause with conservatives on fiscal and regulatory issues.

But these are rules of thumb, as often those we think of as allies on a certain issue abandon us in favor of statism.  Certain conservatives do when they give up capitalism for corporatism.  And Ken at Popehat has a fabulous example of a situation -- protection of defendant's rights in a trial -- where expected liberal support does not materialize.   He discusses the case of an accused child pornography creator

In real life, the accused has the right to review the evidence against him. But how you feel about that scenario can help to determine whether you lean liberal orlibertarian — whether you are suspicious of state power in all instances, or whether you trust the state and look to its firm hand when it comes to hot-button issues, like OMG THINK OF THE CHILDREN!

We’ve been conditioned by the culture to expect that “liberal” and “supportive of due process and fundamental fairness to all people accused of crimes” go hand-in-hand. It’s a lie.

I think the same holds true in other circumstances, like rape trials.  Rights liberals would normally fight for, say, in the case of an accused black murderer in Mississippi were totally tossed aside for white college students accused of rape at Duke.

There are certain regulatory agencies where it is clear from the outset that most of the agency's activity is merely aimed at protecting their own jobs and power.

The one such agency I run up against are Alcoholic Beverage Commissions in various states, from whom one must obtain a liquor license.  In the type of small store we run, there are really only two things the state should care about, and even the second is a bit weak

• That we don't sell alcohol to underage kids
• That we don't allow alcohol consumption on the premises

But the liquor licensing process can be interminable.  In Arizona, for example, I have had my applications kicked back to me, which resulted in 2-month delays in the process, because I wrote an address as 1313 48th Pl.  rather than 1313 48th Place.  They spend incredible man-hours looking for nit-picky mistakes like this, and then kick it back so that the whole review process must begin again.  Many states and counties have a second layer of review, to make sure that your new competition is "needed" - after all, we wouldn't want to upset the position of incumbent businesses who are entitled to their market share and who make nice campaign contributions.

Each application has to have a drawing of the store layout and where one plans to put the beer.  If you want to move the beer at a later date, you have to get the state's approval.  (Bizarrely, the drawing in most states has to be by hand -- they will kick back an application with a CAD drawing or architect's drawing).  And don't get me started on the fact I have to be finger-printed by the FBI (so they can be sure I am not Al Capone) before a store I own can sell beer.

All this being said -- and I didn't mean to run on so long but liquor licensing just drives me nuts -- it is nothing to I won't repeat it all, but take this example:

a drug manufacturer must get approval for how much of a drug it plans to produce, as well as the timeframe. If a shortage develops (because, say, the FDA shuts down a competitor’s plant), a drug manufacturer cannot increase its output of that drug without another round of approvals. Nor can it alter its timetable production (producing a shortage drug earlier than planned) without FDA approval.

They have to get their production schedules approved?  What possible justification can there be for this?  But even more outlandish is the apparent drive to regulate drugs that have been on the market for over 70 years and have to date been relatively unregulated because they were on the market before the FDA got its current powers.   Why should a bureaucrat lose her job when there are still unregulated items out there?  Besides, some uneducated American might use these examples of safe, unregulated drugs to question the who regulatory mission!

Several drug shortages (e.g., concentrated morphine sulfate solution, levothyroxine injection) have been precipitated by actual or anticipated action by the FDA as part of the Unapproved Drugs Initiative, which is designed to increase enforcement against drugs that lack FDA approval to be marketed in the United States. (These drugs are commonly called pre-1938 drugs, referring to their availability prior to passage of the Food, Drug, and Cosmetic Act of that year.) Some participants noted that the cost and complexity of completing a New Drug Application (NDA) for those unapproved drugs is a disincentive for entering or maintaining a market presence.

I have heard several medical people joke that it would be tough to get aspirin through the FDA today if it were a new drug and not grandfathered.  Don't know if that is true, but it feels believable.

My son is taking the Spanish AP exam tomorrow and told me on Sunday he needed a cassette tape recorder for the oral part of the exam -- not one of the mini dictation ones but the kind of cassettes you used to use in your car.

Talk about a ubiquitous technology that has all but disappeared in 10 years!  After a lot of looking, thank God for Amazon same day home delivery, I found one they could deliver in time today  (the item gets good reviews, though the most recent review was in 2002!)

Update: Per the comments, fortunately they are providing the tape.

For all the criticism by the Left of corporate corruption, nothing that goes on in even the most dysfunctional corporations matches business as usual budgeting in Washington.  This week in my column at Forbes I present a few vignettes imagining Washington budget logic in a corporate board room.  A sample:

Board Member: Let’s get started.  After an absolutely disastrous year, financially, we’re now five months into our fiscal year and you still have not presented us with a budget for this year.  Why?

CEO Obama: My staff was waiting until their employment contracts were renewed before we presented a budget.

Board Member:  Excuse me?

CEO Obama: You remember — many of my associates in the company had their contracts up for review in November.  They were afraid they might lose their job if you did not like their budget work, so they delayed introducing any budgets until after you renewed their 2-year employment contracts.

Board Member: That seems unbelievably deceptive and feckless.  But let’s leave that aside for a moment.  November was still several months ago, why have we seen no budget since then?

CEO Obama: Well, as you know, I have a number of rivals for my job in this company.  I want to force one of them to suggest a budget first.

Board Member: Why is that?  It seems to me it is your job as leader of this organization to define the budget, particularly given the unprecedented fiscal challenges we face.

CEO Obama:  If I propose a budget first, everyone will just shoot holes in it.  If I let someone else come forward with the budget, I can snipe at it and make my rivals look worse.  In particular, I think that Ryan guy down in Finance may be dumb enough to create a plan.  If he does, I can spend so much time making him look bad you will forget I never submitted a plan of my own.

Spent the weekend playing Spacechem while watching the NCAA basketball tournament.  Though nominally about tearing apart and building molecules, its really a simulation of assembly line design, since you molecular engineering happens mechanically (ie carry atom over here, bond it in reactor, move it over there, etc).  There is a kind of built in re-playability, as most of the puzzles are not that hard to solve in some fashion, but can be very hard to solve efficiently.  For example, the level "No Ordinary Headache" will allow the player up to three reactors, but a one reactor solution is possible.  Took me forever to finally get it. This one is not mine but is not too different from my solution.

To that end, the game provides a distribution curve of other player's solutions based on three stats (number of process cylces required, number of reactors required, number of components required).  Even if you get the puzzle right, you may see you solution was way less efficient than other folks, driving one to try again.  I like this dynamic - it is sort of like duplicate bridge, where one is not judged by just winning the hand, but by how well one scored with the hand vs. other players playing the same hand.

Here is another positive review at South Bend Seven.  And just search "spacechem" in youtube to find zillions of videos of various game solutions, it will give you a feel for the game.

If you want some enjoyable satire this Friday, this is a nice piece from Ken at Popehat, who has gone the libel tourism route by using French courts to sue a US editor for a bad review of a US book by a US author.

The article becomes all the sweeter as a recent email he received raises the possibility that this particular academic completely missed the heavy-handed irony and satire.

From PERC, a group focused on free-market environmentalism

The Julian Simon Fellowship has an open application process, so applications are always welcome. Review of applications will begin as soon as they are received.

The Julian Simon Fellowship is one of the nation's most prestigious opportunities for scholars to develop policy-oriented research on natural resource and environmental conservation. The in-residence fellowship is intended to continue the legacy of the late Julian Simon, whose research led to a massive re-evaluation by scholars and policy makers of their views on the interplay between population, natural resources, and the environment.

The ideal candidate for this fellowship is someone like Julian; an excellent scholar with a focus on empirical work and an imaginative research agenda that emphasizes natural resource and environmental issues.

Each Julian Simon Fellow is expected to spend at least two months in residence at PERC developing a paper of publishable quality, one that has significant policy implications. During their stay at PERC, Julian Simon Fellows are expected to present a seminar on their work.

Each Julian Simon Fellow will receive an honorarium of $20,000 plus office space, office support, and a congenial, stimulating work environment. Fellows will be responsible for their accommodations in Bozeman, but PERC is happy to assist with arrangements.

I want to post more later when I have time on the whole notion of giving the US President an Internet kill switch (short review:  yuk!)

But I wanted to correct one bit of sloppiness in a number of posts.  Access to the Internet is not a human right.  If it were, the implication is that some groups of people would have to be coerced to provide Internet access to those who currently don't enjoy it.

The correct way to phrase the issue is "Turning off or blocking the Internet is not a government right."

• Migrated about 20 web sites to my new server (actual a virtual private server rather than a dedicated server, but it seems to have most of the functionality of dedicated at a lower price -- performance remains to be tested).  This was sort of a death march as it was incredibly dull and repetitive, especially since many of the sites use WordPress as the content management system so they required database setup and migration as well.  Basically got almost everything done except this site.  I am sure after 20 smooth moves Murphy's Law will cut in on the largest and most complicated.
• Created our Christmas / Holiday card.  Some 20 years ago I set the unfortunate precedent of trying to do something unique for our cards, so I have made this a double extra more time consuming process than it has to be.  (past examples here, here, here)
• Made a lot of progress laying track on my model railroad.  All my track is scratch built (from rails and ties) and so it takes a while, but I have nearly all the major switches in place, which are the real time consumers when hand laying track
• Created a second RAID for my home theater system.  Incredibly, the original 8Tb raid (5x2 TB drives in a RAID 5) is almost full.  Chalk this up in part to Blu Ray rips (which can be 30Gb each) but also to my finally ripping TV series I have on disk (Sopranos, Mad Men, Firefly, etc).  These involve a lot of disks.

At some point soon I want to write a review of my experience with the new SageTV version 7.0 software, which is an ENORMOUS improvement over their old versions.  The Sage system is still for advanced users, but the process for managing plugins and extensions (the whole point of Sage is its customizability) is greatly improved.  The new HD300 set top box is also improved, though with a flaw or two.  You are welcome to email me if you are considering Sage (or if you want something more capable than most media streaming boxes) and I can give you the pros and cons.

Now all I need is a few Christmas present ideas for my wife.

Today's word in question:  "safe"

The Environmental Protection Agency is holding public hearings today to review a proposed safe exposure limit for dioxin, a known carcinogen and endocrine disruptor produced as a common industrial byproduct.

It's all but impossible to avoid exposure to dioxin. Research done by the Environmental Working Group has shown that adults are exposed to 1,200 times more dioxin than the EPA is calling safe "” mostly through eating meat, dairy and shellfish "” and mothers pass it on to babies in the womb and in breast milk. A nursing infant ingests an amount 77 times higher than what the EPA has proposed as safe exposure. (Formula is also widely contaminated with the stuff.)

If you tell me that despite falling cancer incidence and survival rates and longer life-spans, we are all exposed to a chemical at 1200x its "safe" level, I might argue that we have defined the safe level too low.  Of course, the author draws just the opposite conclusion, arguing the standard needs to be tightened.

Two observations

1. Things are getting better.  Apparently dioxin emissions (mostly from burning trash) have fallen by 90+% over the last twenty years.  In the blog post above, the author lambastes the EPA for dragging its feet on this standard for 30 years, but the lack of it sure does not seem to have been a problem

   

2. I am not sure how setting a dioxin standard by the EPA is going to help.  Since most dioxin makes its way into the food chain (such as into dairy products), I suppose this would then give the government license to pound dairy farmers for the dioxin content of their products.  But what does this get us, and how is this the dairy farmers' fault?  For the last 30 years, as described at this site, the EPA and voluntary efforts by emitters have been working step by step through the pie chart above, knocking off the worst emitters.   You can see that clearly in the change of mix and the overall reduction.  This seems like a smart strategy.

One of my theories I have mentioned before on this blog is that the worst abuses of freedom occur when the Left and Right in this country agree.  Here is another great example -- combine the Right's law-and-order drive to hand more power to,  and remove accountability from, police and prosecutors with the Left's need to string up some executives after the Enron collapse -- and you get this:

The DOJ has inexplicably teed up another trial of Brown, who was the only one of the Merrill defendants who was convicted on additional charges of perjury and obstruction of justice for having the temerity of protesting his innocence to the grand jury that originally investigated the Nigerian Barge deal. Brown's new trial is currently scheduled to begin on September 20.

But in the meantime, Brown's legal team has been leafing through enormous amounts of exculpatory evidence that the Enron Task Force withheld from the Merrill defendants in connection with the first trial back in 2005, but which the DOJ has recently been forced to disclose.

The result of the Brown team's effort is set forth below in the Supplemental Memorandum in support of a motion for a new trial for Brown on the perjury and obstruction charges (the downloaded version of the memo is bookmarked in Adobe Acrobat to facilitate ease of review). The memorandum details the appalling length that the Enron Task Force went during the first trial in suppressing exculpatory evidence in favor of Brown and his co-defendants and generally disregarding the rule of law in order to obtain convictions. As the memorandum concludes:

The conclusion is now inescapable that the ETF engaged in a calculated, multi-step process to deprive Brown of his constitutional right to Due Process. (1) They repeatedly denied the existence of Brady material, told this court they had met their Brady obligations and fought vehemently against producing anything [exhibit reference and footnote omitted]. They highlighted only selected material in a veritable garden of Brady evidence "“ much of their selections being vague, tangential or marginal"“while working around clear, declarative, relevant exculpatory material even in the same page, paragraph or document. (3) When ordered by the Court to produce summaries to the defense, they further redacted even the Brady material they had themselves highlighted and withheld the crucial facts that they had highlighted as Brady. (4) They egregiously capitalized on their misconduct at trial by making assertions that were directly belied by the exculpatory evidence they withheld.  .  .  .

The memorandum goes on to set out dozens of Brady violations, including charts that compare the exculpatory statements that the Enron Task Force withheld prior to the first trial with the incriminating statements that the Enron Task Force extracted from witnesses during that trial.

Folks, this is really bad stuff. But as bad as it is, I have not seen any mention of it in the mainstream media.

Remember all that BS about the Obama administration only being ruled by facts and science?  This is a mythology at the core of the progressive movement, that it is possible to have a wise dictator who uses the heavy hand of government coercion only for the best interests of the country, driven only by science and not by political influence.

This is of course a crock.  It was a popular point of view in the early 20th century, and at the heart of efforts like Mussolini's fascism, which in turn was much admired by FDR and emulated in US efforts like the NRA (the blue eagle, not the gun organization).  Over time, history has demonstrated folks like Hayek right on the knowlege problem (no one can possibly be smart enough to make optimum decisions for everyone, particularly when everyone has different preferences) while we have plenty of evidence to demonstrate the incentives for politicians are skewed so badly as to make good decisions almost impossible.

But the myth persists, even in the face of obvious counter-examples, like this (emphasis added):

The economic report released last week by Health and Human Services, which indicated that President Barack Obama's health care "reform" law would actually increase the cost of health care and impose higher costs on consumers, had been submitted to the office of HHS Secretary Kathleen Sebelius more than a week before the Congressional votes on the bill, according to career HHS sources, who added that Sebelius's staff refused to review the document before the vote was taken."The reason we were given was that they did not want to influence the vote," says an HHS source. "Which is actually the point of having a review like this, you would think."

The analysis, performed by Medicare's Office of the Actuary, which in the past has been identified as a "nonpolitical" office, set off alarm bells when submitted. "We know a copy was sent to the White House via their legislative affairs staff," says the HHS staffer, "and there were a number of meetings here almost right after the analysis was submitted to the secretary's office. Everyone went into lockdown, and people here were too scared to go public with the report."

In the end, the report was released several weeks after the vote -- the review by the secretary's office reportedly took less than three days -- and bore a note that the analysis was not the official position of the Obama administration.

Wouldn't want to influence a vote with actual facts.

From the Telegraph, via  Q&O:

Barack Obama's administration has authorised the assassination of the radical Muslim cleric Anwar al-Awlaki, a rare move against an American citizen.

I am reminded of what I wrote on the day of Obama's inauguration:

I will be suitably thrilled if the Obama administration renounces some of the creeping executive power grabs of the last 16 years, but he has been oddly silent about this.  It seems that creeping executive power is a lot more worrisome when someone else is in power.

But don't worry, separation of powers has been respected:

The decision to add him to the US hit list required a National Security Council review because of his citizenship.

You see, before Obama can unilaterally order an American citizen killed, he has to review the decision with, uh, a group of people he appointed and that work directly for him.  From Bruce McQuain:

But who the hell is Barack Obama to arbitrarily and unilaterally waive Constitutional due process (oh, that's right, he's a Constitutional law professor, isn't he?) and order the assassination of a US citizen?  And as an aside "“ where are all the liberal voices who spent every waking hour worrying about George Bush's eavesdropping and loudly denouncing it, forever and ever, amen?  Why are they, for the most part, silent on the subject of assassinating a US citizen?

Every study I have ever seen has said that corn ethanol is only marginally energy-positive when its growing and production costs are considered and barely breakeven on CO2.  In other words, it costs a lot and does nothing, even before one considers negative effects to food prices and land use.

So of course, the Obama administration may soon demand that we subsidize more of it

Burdened by falling gasoline consumption and excess production capacity, ethanol producers appealed to the government on Friday to raise the 10 percent limit on ethanol in most gasoline blends to as high as 15 percent.

Ethanol plants are closing across the country and some ethanol producers are declaring bankruptcy. The appeal will require the Obama administration to decide whether to increase federal support for the industry, which has already benefited from an array of subsidies, tax credits and Congressional production mandates.

"Approving the use of ethanol blends up to 15 percent is a necessary and positive step," said Bob Dinneen, president of the Renewable Fuels Association, an industry lobbying group, "to ensure the full potential of a robust domestic ethanol industry."

The Environmental Protection Agency and the Energy Department have been testing higher ethanol blends. The E.P.A. has nine months to review the request, but it could decide before that to increase the blend cap slightly, to 12 or 13 percent.

Energy Secretary Steven Chu has indicated that he would favor at least a small increase in ethanol levels unless auto companies said there was a risk the change would damage their products.

At least the article is marginally honest - its starts with the true reason for the mandate - improving the bottom line of favored businesses, not energy or environmental policy.  Chu seems to be joining Krugman as another Nobel prize winner turned political hack.  In the past I have had Chu's supposed gravitas thrown at me in climate debates -- I think this should settle just how Chu makes choices between what science tells him vs. what politcal pressures are demanding.

Government bureaucracies do not exercise power by allowing activities to occur - they only have power, and thus have reason to justify their continued funding and jobs, when they say no.   Every incentive that they have is to say no.  When a government agency allows progress to proceed smoothly, it is doing so because some person or small group is fighting against the very nature of the organization.  Anyone who believes otherwise about government agencies is challenged to go build and open a new restaurant in Ventura County, California.  Here is the latest example:

The [weatherizing] program was a hallmark of the American Recovery and Reinvestment Act, a way to shore up the economy while encouraging people to conserve energy at home. But government rules about how to run what was deemed to be a ''shovel-ready'' project, including how much to pay contractors and how to protect historic homes during renovations, have thwarted chances at early success, according to an Associated Press review of the program.

''It seems like every day there is a new wrench in the works that keeps us from moving ahead,'' said program manager Joanne Chappell-Theunissen. She has spent the past several months mailing in photographs of old houses in rural Michigan to meet federal historic preservation rules. ''We keep playing catch-up.''

And of course, even in a skeptical article about a "stimulus" project, no one ever mentioned what productive activities the $5 billion was being used for by private individuals before the government yanked it away for this little catastrophe.

By the way, the overblown rhetoric award has to go to this:

''This is the beginning of the next industrial revolution with the explosion of clean energy investments,'' said assistant U.S. Energy Secretary Cathy Zoi. ''These are good jobs that are here to stay.''

Given that the first one was about steel mills and railroads and oil and electricity, if this new industrial revolution is all about caulking, I think I am getting nostalgic for the first one.

Over the last several years, we have been replacing many of the full-sized pickups we use in our campground business with mini-trucks from Japan.  They are cheaper to insure, cheaper to buy, easy to repair, and get about 60 miles to the gallon.  We typically buy them used in container-loads of six or seven, and we used to get them for less than $10,000 a container -- now they cost almost this much individually.

This year the prices have sky-rocketed, and they have been hard to find.  I finally discovered the reason.  It seems the EPA has halted their importation.  These are trucks that are from an emissions regime (in Japan) harsher than ours and that have three times the gas mileage of the trucks they are replacing.  But apparently the EPA doesn't have rules for them and doesn't know how to categorize them, and anything a bureaucrat doesn't have rules for must be illegal, right?  So now we are forced to go back to full-size pickup truck purchases until the EPA can catch up with the market.

Update: Apparently the EPA is going to review these trucks model by model.  This is so stupid.  They need some kind of class waiver.

From the TaxProf:

President Obama today issued a presidential memorandum directing the Office of Management and Budget, the Treasury Department, and other federal agencies to block contractors who are delinquent on their taxes from receiving new government contracts. The memorandum also directs the IRS to review the accuracy of companies' tax delinquency claims and asks Congress to enact enforecement tools.

This administration continues to follow the consistent principle that taxes should be applied ruthlessly to out-of-favor and unsympathetic groups, and forgiven or exempted for friends of the Administration.  Does anyone else find it odd that our first black President is doing so much to undermine the equal protection clause of the 14th Amendment?

Hopefully this is true, but it appears that Google is fed up with Chinese hijinx and is considering either pulling out of the country or insisting on being more open and less filtered.  I have given Google a lot of grief here for enabling Chinese censorship, so kudos if they are starting to rethink their relationship with China.

These attacks and the surveillance they have uncovered"“combined with the attempts over the past year to further limit free speech on the web"“have led us to conclude that we should review the feasibility of our business operations in China. We have decided we are no longer willing to continue censoring our results on Google.cn, and so over the next few weeks we will be discussing with the Chinese government the basis on which we could operate an unfiltered search engine within the law, if at all. We recognize that this may well mean having to shut down Google.cn, and potentially our offices in China.

I thought this was pretty good.  I was so focused on the Dances with Wolves parallels that I missed this one.    Via the South Bend Seven, who has other review notes on Avatar.

When someone starts to shout "but its in the peer-reviewed literature" as an argument-ender to me, I usually respond that peer review is not the finish line, meaning that the science of some particular point is settled.  It is merely the starting point, where now a proposition is in the public domain and can be checked and verified and replicated and criticized and potentially disproved or modified.

The CRU scandal should, in my mind, be taken exactly the same way.  Unlike what more fire-breathing skeptics have been saying, this is not the final nail in the coffin of catastrophic man-made global warming theory.  It is merely a starting point, a chance to finally move government funded data and computer code into the public domain where it has always belonged, and start tearing it down or confirming it.

To this end, I would like to share a post from year ago, showing the kind of contortions that skeptics have been going through for years to demonstrate that there appear to be problems in  key data models -- contortions and questions that could have been answered in hours rather than years if the climate scientists hadn't been so afraid of scrutiny and kept their inner workings secret.  This post is from July, 2007.  It is not one of my most core complaints with global warming alarmists, as I think the Earth has indeed warmed over the last 150 years, though perhaps by less than the current metrics say.  But I think some folks are confused why simple averages of global temperatures can be subject to hijinx.  The answer is that the averages are not simple:

A few posts back, I showed how nearly 85% of the reported warming in the US over the last century is actually due to adjustments and added fudge-factors by scientists rather than actual measured higher temperatures.  I want to discuss some further analysis Steve McIntyre has done on these adjustments, but first I want to offer a brief analogy.

Let's say you had two compasses to help you find north, but the compasses are reading incorrectly.  After some investigation, you find that one of the compasses is located next to a strong magnet, which you have good reason to believe is strongly biasing that compass's readings.  In response, would you

1. Average the results of the two compasses and use this mean to guide you, or
2. Ignore the output of the poorly sited compass and rely solely on the other unbiased compass?

Most of us would quite rationally choose #2.  However, Steve McIntyre shows us a situation involving two temperature stations in the USHCN network in which government researchers apparently have gone with solution #1.  Here is the situation:

He compares the USHCN station at the Grand Canyon (which appears to be a good rural setting) with the Tucson USHCN station I documented here, located in a parking lot in the center of a rapidly growing million person city.   Unsurprisingly, the Tucson data shows lots of warming and the Grand Canyon data shows none.  So how might you correct Tucson and the Grand Canyon data, assuming they should be seeing about the same amount of warming?  Would you

average them, effectively adjusting the two temperature readings

towards each other, or would you assume the Grand Canyon data is cleaner

with fewer biases and adjust Tucson only?   Is there anyone who would not choose the second option, as with the compasses?

The GISS data set, created by the Goddard Center of NASA, takes the USHCN data set and somehow uses nearby stations to correct for anomalous stations.  I say somehow, because, incredibly, these government scientists, whose research is funded by taxpayers and is being used to make major policy decisions, refuse to release their algorithms or methodology details publicly. They keep it all secret!  Their adjustments are a big black box that none of us are allowed to look into  (and remember, these adjustments account for the vast majority of reported warming in the last century).

We can, however, reverse engineer some of these adjustments, and McIntyre does.  What he finds is that the GISS appears to be averaging the good and bad compass, rather than throwing out or adjusting only the biased reading.  You can see this below.  First, here are the USHCN data for these two stations with only the Time of Observation adjustment made (more on what these adjustments are in this article).

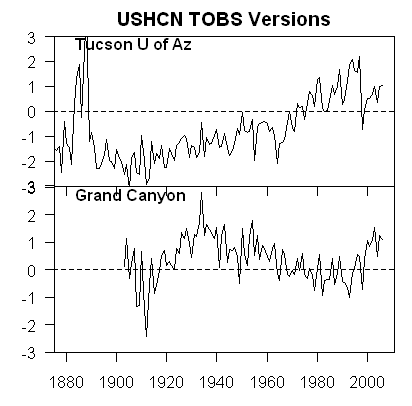
As I said above, no real surprise "“ little warming out in undeveloped nature, lots of warming in a large and rapidly growing modern city.  Now, here is the same data after the GISS has adjusted it:

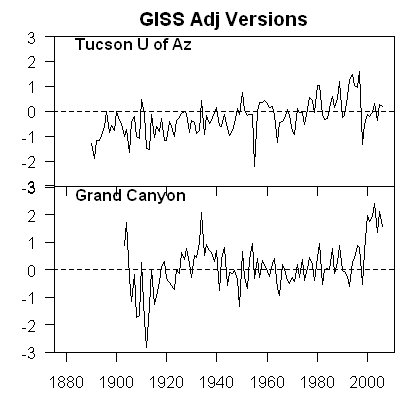
You can see that Tucson has been adjusted down a degree or two, but Grand Canyon has been adjusted up a degree or two (with the earlier mid-century spike adjusted down).  OK, so it makes sense that Tucson has been adjusted down, though there is a very good argument to be made that it should be been adjusted down more, say by at least 3 degrees**.  But why does the Grand Canyon need to be adjusted up by about a degree and a half?  What is biasing it colder by 1.5 degrees, which is a lot?  The answer:  Nothing.  The explanation:  Obviously, the GISS is doing some sort of averaging, which is bringing the Grand Canyon and Tucson from each end closer to a mean.

This is clearly wrong, like averaging the two compasses.  You don't average a measurement known to be of good quality with one known to be biased.  The Grand Canyon should be held about the same, and Tucson adjusted down even more toward it, or else thrown out.  Lets look at two cases.  In one, we will use the GISS approach to combine these two stations"“ this adds 1.5 degrees to GC and subtracts 1.5 degrees from Tucson.  In the second, we will take an approach that applies all the adjustment to just the biases (Tucson station) "” this would add 0 degrees to GC and subtract 3 degrees from Tucson.  The first approach, used by the GISS, results in a mean warming in these two stations that is 1.5 degrees higher than the more logical second approach. No wonder the GISS produces the highest historical global warming estimates of any source!  Steve McIntyre has much more.

** I got to three degrees by applying all of the adjustments for GC and Tucson to Tucson.  Here is another way to get to about this amount.   We know from studies that urban heat islands can add 8-10 degrees to nighttime urban temperatures over surrounding undeveloped land.  Assuming no daytime effect, which is conservative, we might conclude that 8-10 degrees at night adds about 3 degrees to the entire 24-hour average.

Postscript: Steve McIntyre comments (bold added):

These adjustments are supposed to adjust for station moves "“ the procedure is described in Karl and Williams 1988 [check], but, like so many climate recipes, is a complicated statistical procedure that is not based on statistical procedures known off the island. (That's not to say that the procedures are necessarily wrong, just that the properties of the procedure are not known to statistical civilization.) When I see this particular outcome of the Karl methodology, my mpression is that, net of the pea moving under the thimble, the Grand Canyon values are being blended up and the Tucson values are being blended down. So that while the methodology purports to adjust for station moves, I'm not convinced that the methodology can successfully estimate ex post the impact of numerous station moves and my guess is that it ends up constructing a kind of blended average.

LOL.  McIntyre, by the way, is the same gentleman who helped call foul on the Mann hockey stick for bad statistical procedure.

Just what we need, the government choosing winners and losers in media like they do earmark recipients.  Since government ownership of GM was politicized in Congress before the ink on the court agreements was dry, I wonder how fast Congress will find a way to use a government media bailout to punish the critical and reward sycophants.

A top Democratic lawmaker predicted on Wednesday that the government will be involved in shaping the future for struggling U.S. media organizations.House Energy and Commerce Committee Chairman Henry Waxman, saying quality journalism was essential to U.S. democracy, said eventually government would have to help resolve the problems caused by a failing business model.

Waxman, other U.S. lawmakers and regulators are looking into various options to help a newspaper industry hurt by the shift in advertising revenues to online platforms.

Waxman continues:

"Eventually government is going to have to be responsible to help and resolve these issues,"

Why?  You mean like when the US government stepped up in the 19th century to bail out pamphleteers and failing broadsheet publishers when the market moved to new media?  Or when it moved to bail out network television under assault from new cable channels?  Remember that?  Neither do I.

Next steps:

At the Federal Communications Commission, officials are embarking on a quadrennial review of the state of U.S. media. The study, which is mandated by Congress, seeks to determine whether current rules should be changed to allow for a more vibrant media industry serving a diverse audience.

We have that.  Its called the Internet.  It emerged entirely free of government action (save some funding of some original infrastructure).  Go away.

This is from a post of mine last January, long before the Climategate scandal  (though most of us who spent a lot of time with climate issues knew of the Climategate abuses long before the smoking gun emails were found).  The one thing this article does not mention is what we know today -- that climate scientists were actively working to keep skeptical studies out of the literature, even to the point of getting editors fired.

Peer review is not a guarantee of accuracy or a good housekeeping seal of correctness.  It is a process that insures a work is worthy of publication by a scholarly magazine.  Whether a scientific question is "settled" does not end with peer review, it only begins. It becomes settled after it survives decades of criticism and replication work, a process that was stonewalled by the folks at the CRU, which is really the heart of the scandal.

Cafe Hayek has a series of articles here and here on the absurd numerical games going on to pump up the stimulus numbers (which I also offered example of here, where pay increases were considered a "job saved").

For example:

Up to one-fourth of the 110,000 jobs reported as saved by federal stimulus money in California probably never were in danger, a Bee review has found.

California State University officials reported late last week that they saved more jobs with stimulus money than the number of jobs saved in Texas "“ and in 44 other states.

In a required state report to the federal government, the university system said the $268.5 million it received in stimulus funding through October allowed it to retain 26,156 employees.

That total represents more than half of CSU's statewide work force. However, university officials confirmed Thursday that half their workers were not going to be laid off without the stimulus dollars.

"This is not really a real number of people," CSU spokeswoman Clara Potes-Fellow said. "It's like a budget number."

Also here:

While Massachusetts recipients of federal stimulus money collectively report 12,374 jobs saved or created, a Globe review shows that number is wildly exaggerated. Organizations that received stimulus money miscounted jobs, filed erroneous figures, or claimed jobs for work that has not yet started.

The Globe's finding is based on the federal government's just-released accounts of stimulus spending at the end of October. It lists the nearly $4 billion in stimulus awards made to an array of Massachusetts government agencies, universities, hospitals, private businesses, and nonprofit organizations, and notes how many jobs each created or saved.

But in interviews with recipients, the Globe found that several openly acknowledged creating far fewer jobs than they have been credited for.

One of the largest reported jobs figures comes from Bridgewater State College, which is listed as using $77,181 in stimulus money for 160 full-time work-study jobs for students. But Bridgewater State spokesman Bryan Baldwin said the college made a mistake and the actual number of new jobs was "almost nothing.'' Bridgewater has submitted a correction, but it is not yet reflected in the report.

It is becoming clearer and clearer that the vast majority of "jobs saved" were in government, and in effect the stimulus merely had the effect of bailing out state governments that were able to use stimulus money to put off their budget reckonings.